Planning tool
Year levels
Strands
Expected level of development
Australian Curriculum Mathematics V9: AC9M6N08, AC9M5N09
Numeracy Progression: Additive strategies: P8, Multiplicative strategies: P6, Understanding money: P7
At this level, students use mathematical modelling to solve practical problems involving additive and multiplicative situations including in financial contexts. They formulate the problem, choose operations and efficient calculation strategies, and use digital tools where appropriate. They interpret and communicate solutions in terms of the situation, justifying the choices made.
The mathematical modelling process includes identifying and solving a problem that has a real-world context or is presented as a scenario for students to investigate the possible outcomes applying their knowledge and understanding of mathematics concepts, structures and relationships and their mathematical thinking reasoning and problem-solving skills.
Present contexts that enable students to apply mathematical thinking and approaches of their choosing. Resist the temptation to provide a ready-made path with the relevant mathematical operations as students’ thinking in these situations may be limited. Instead, use enabling prompts, questioning and feedback to guide students through the process. Use resources that have relevant problems to solve, however, do not include the scaffolding and allow students to choose which mathematical operations to use.
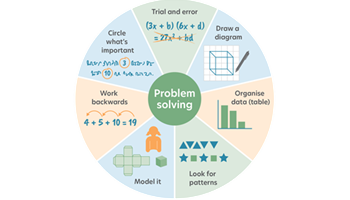
Use the framework for mathematical modelling that provides seven steps to solve a real-world problem or scenario.
Help students develop their computational thinking skills by breaking down a problem and working out what mathematical operations and computations they need to make. Students may employ a range of mathematics skills depending on the richness of the problem.
Look for opportunities to highlight different approaches students’ use and encourage a discussion about the range of approaches and the choices behind them.
Teaching and learning summary:
- Provide guidance on using a mathematical modelling process to solve real-world problems.
- Generate problems for students that encourage them to choose relevant mathematical operations and efficient calculation strategies.
- Explicitly teach and provide guidance to support students to fluently solve calculations as the opportunity arises.
- Encourage students to make estimates for solutions involving rational numbers and percentages to check the reasonableness of solutions.

Students:
- use additive and multiplicative thinking to solve mathematical problems
- use appropriate estimation strategies
- model practical situations choosing an additive/multiplicative model and use a number sentence to represent it
- using physical materials, arrays and bar models to assist with calculations
- choose and evaluate calculation strategies in terms of efficiency and solutions in terms of reasonableness
- communicate findings of their investigations in the context of the problem situation.
Some students may:
- not yet be able to choose the correct operation to solve a mathematical problem. Often, we suggest an operation to solve a number problem or provide scaffolding to the extent that students are not given an opportunity to think about how to solve a problem. Prompt student thinking using questioning and encourage different ways to solve problems.
- require support to evaluate and explain their choices. Often students focus on finding a solution without justification for this choice. Help students to slow down and think about whether the choices they made were effective or not, and why.
The Learning from home activities are designed to be used flexibly by teachers, parents and carers, as well as the students themselves. They can be used in a number of ways including to consolidate and extend learning done at school or for home schooling.
Learning intention
- We are learning to choose relevant approaches and mathematical strategies to solve problems.
Why are we learning about this?
- We solve maths problems everyday in life and work.
What to do
The bathroom in Adam’s house has a leaking pipe. He realises there is an issue with the plumbing so he has contacted three plumbers to see if they can help him figure out what is going on. Here is some information from each plumber:
| Plumber 1 | Plumber 2 | Plumber 3 | |
| Callout fee | Nil | $50 | $80 |
| First 3 hours | $500 | $330 | $420 |
| Every additional hour | $85 | $70 | $50 |
| Unexpected additional costs | $100 | $50 | $50 |
- Which plumber should Adam choose? List all the possibilities and show your calculations.
- Why should Adam choose this plumber?
- Is there a plumber who would be ideal for quick jobs? Is there another plumber who would be better to call in in the event of extended works to the plumbing?
- How might Adam save some money?
Success criteria
I can:
- select an approach to solve a practical problem
- use mathematical modelling to specify the mathematical problem and then formulate and solve the problem
- evaluate my approach (calculation strategies used) and my solution in terms of the problem.
Please note: This site contains links to websites not controlled by the Australian Government or ESA. More information here.
Teaching strategies
A collection of evidence-based teaching strategies applicable to this topic. Note we have not included an exhaustive list and acknowledge that some strategies such as differentiation apply to all topics. The selected teaching strategies are suggested as particularly relevant, however you may decide to include other strategies as well.
-
Classroom talks
Classroom talks enable students to develop language, build mathematical thinking skills and create mathematical meaning through collaborative conversations.
Go to resource -
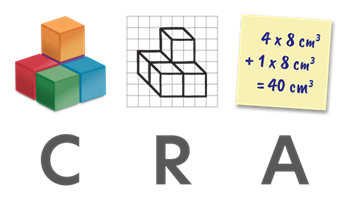
Concrete, Representational, Abstract (CRA model)
The CRA model is a three-phased approach where students move from concrete or virtual manipulatives, to making visual representations and on to using symbolic notation.
Go to resource -
Mathematics investigation
By giving students meaningful problems to solve they are engaged and can apply their learning, thereby deepening their understanding.
Go to resource -
Multiple exposures
Providing students with multiple opportunities within different contexts to practise skills and apply concepts allows them to consolidate and deepen their understanding.
Go to resource -

Questioning
A culture of questioning should be encouraged and students should be comfortable to ask for clarification when they do not understand.
Go to resource -
Feedback
It has been shown that good feedback can make a significant difference to a student’s future performance.
Go to resource -
Metacognitive strategies
Metacognitive skills are those that students need to be able to reflect on their own learning, set goals for themselves, monitor their progress and make improvements to move forward.
Go to resource
Teaching resources
A range of resources to support you to build your student's understanding of these concepts, their skills and procedures. The resources incorporate a variety of teaching strategies.
-

Mathematical Modelling: A guidebook for teachers and teams
Use this guide to familiarise yourself with the framework suggested to introduce mathematical modelling.
Go to resource -
The deca tree
This task can be used to explore and use mathematical modelling to solve a problem that engages multiplicative thinking.
Go to resource -

Counting trees
Students devise a method for estimating the numbers of trees in a plantation then use their method to estimate the number of two different kinds of tree.
Go to resource -

Owning a pet
Students learn about pet ownership in Australia and the cost of buying and keeping a pet.
Go to resource -

Paying It Forward Years 5-6
In this unit students will understand how taxation is collected and how it is spent responsibly to provide for all members of the community.
Go to resource -
Tasty treats
This is a level 5 number link activity from the Figure It Out series. It is focused on multiplying fractions by 2 and by 10, as well as multiplying and dividing with money. A PDF of the student activity is included.
Go to resource